Kai Yang and Hongwei Zhang
kyang@mie.eng.wayne.edu
This is our first research paper in comparisons of TRIZ and Axiomatic Design. In this paper, Axiomatic Design (AD) and TRIZ are briefly reviewed and their possible relationships are analyzed and listed.
It is self-evident that decisions made during design stage of product and process development profoundly affect the product quality and productivity. Traditionally, product and process have been designed based on know-how and trail-and-error; however the empiricism of a designer is limited and can lead to costly mistakes. Axiomatic Design and The Theory of Inventive Problem Solving (TRIZ) have been developed to aid design decision making and related problem solving.
Axiomatic design is a general methodology that helps designers to structure and understand design problems, thereby facilitating the synthesis and analysis of suitable design requirements, solutions, and processes. This approach also provides a consistent framework from which the metrics of design alternatives can be quantified.
TRIZ offers a wide-ranging series of tools to help designers and inventors to avoid trial-and-error approach in design process and to solve problem in creative and powerful ways. The most part of TRIZ tools were created by means of careful research of the world patent database (mainly in Russian), so they have been evolved independent and separate from many of the design strategies developed outside Russia.
The design process usually consists of several steps as follows [1] [3] [8].
· Establish design objectives to satisfy a given set of customer attributes
· Generate ideas to create plausible solutions
· Analyze the solution alternatives that best satisfies the design objectives
· Implement the selected design
Decisions made during the each step of design process will profoundly affect product quality and manufacturing productivity. To aid design decision making, Axiomatic Design theory has been developed in the last decade. The Axiomatic Design approach to the execution of the above activities is based on the following key concepts:
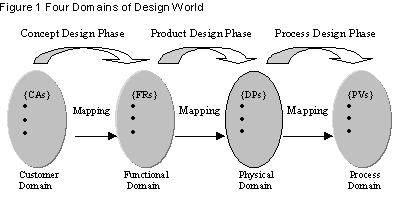
(1) There exist four domains in the design world, customer domain, functional domain, physical domain and process domain. The needs of the customer are identified in customer domain and are stated in the form of required functionality of a product in functional domain. Design parameters that satisfy the functional requirements are defined in physical domain, and in process domain manufacturing variables define how the product will be produced. The whole design process involves the continuous processing of information between and within four distinct domains.
(2) Solution alternatives are created by mapping the requirements specified in one domain to a set of characteristic parameters in an adjacent domain. The mapping between the customer and functional domains is defined as concept design; the mapping between functional and physical domains is product design; the mapping between the physical and process domains corresponds to process design.
(3) The mapping process can be mathematically expressed in terms of the characteristic vectors that define the design goals and design solution.
(4) The output of each domain evolves from abstract concepts to detailed information in a top-down or hierarchical manner. Hierarchical decomposition in one domain cannot be performed independently of the other domains, i.e., decomposition follows zigzagging mapping between adjacent domains.
(5) Two design axioms provide a rational basis for evaluation of proposed solution alternatives and the subsequence selection of the best alternative. The two axioms can be stated as follows:
Axiom 1 (independence axiom): maintain the independence of the FRs.
Axiom 2 (information axiom): minimize the information content of the design.
The first axiom is the independent axiom, and it focus on the nature of the mapping between “what is required” (FRs) and “how to achieve it” (DPs). It states that a good design maintains the independence of the functional requirements. The second axiom is the information axiom and it establishes information content as a relative measure for evaluating and comparing alternative solutions that satisfy the independence axiom.
The four-domain structure is schematically illustrated in figure 1. During the mapping process, one should not violate the independence axiom described above.
In the product design, the creation or synthesis phase of design involves mapping the FRs in the functional domain to design parameters (DPs) in the physical domain. Since the complexity of the solution process necessarily increases with the number of FRs, it is important to describe the perceived design needs in terms of a minimum set of independent requirements. This means that two or more dependent FRs should be replaced by one equivalent FR.
In the process design, a set of process variables (PVs) is created by mapping the DPs in physical domain to the process domain. The PVs specify the manufacturing methods that produce the DPs.

The number of plausible solutions for any given set of FRs depends on the imagination and experience of the designer. Thus, the design axioms are used to determine acceptable design solution. Defining {FR} as a vector of functional requirements and {DP} as a corresponding vector of design parameters, and {PV} as vector of process variables, the mapping between the functional and physical domains, between physical and process domains can be expressed mathematically in equation (1) and (2).
In equation (1) and (2), [A] and [B] are called design matrix. To satisfy the Independence Axiom, matrix [A] and [B] must be either diagonal or triangular. When the design matrix, for example [A], is diagonal, each of the FR can be satisfied independently by means of one DP and this design is an uncoupled design. When the design matrix is triangular, the independence of FRs can guarantee if the DPs are changed in a proper sequence, and this design is a decoupled design. When there are many FR&DP, two quantitative measures, reangularity and semangularity in equation (3) and (4), are used to determine the independence of the functional requirements [1].

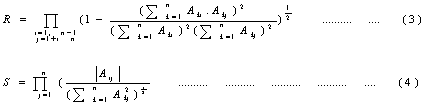
A design’s information content is calculated according to the following logarithmic expression.
![]()
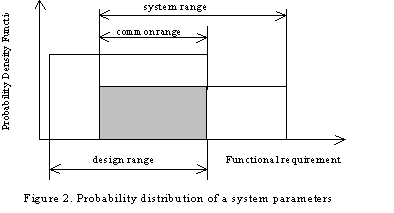
Where, P is the probability of successfully satisfying the functional requirements. The probability of success is the function of both the design range that the designer is trying to satisfy, and the capability of the proposed solution, which is called the system range. A desirable solution corresponds to the region of overlap between the design range and the system range shown in figure 2 (for uniform probability function). The region of overlap is called the common range. Then the definition for the information content given by equation (5) can be rewritten as in equation (6). When there are n functional requirements, the total information is given by equation (7).
![]() (6)
(6)
![]()
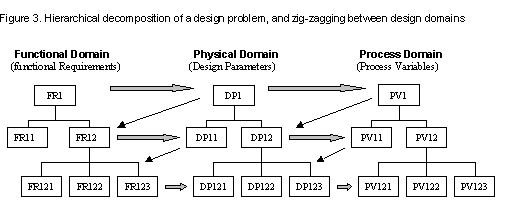
Figure 3 is a graphic interpretation of the general mapping process between functional and physical domains, and between physical and process domains. The FR-to-DP mapping takes place over a number of levels of abstraction. A given set of FRs must be successfully mapped to a set of DPs in the physical domain prior to the decomposition of the FRs. Iterations between FR-to-DP mapping and the functional decomposition suggest a zigzagging between the functional and physical domains.
TRIZ is Russian acronym for The Theory of Inventive Problem Solving that originated from extensive studies of technical and patent information. Studies of patent collections by Altshuller, the founder of TRIZ, indicated that only one per cent of solutions was truly pioneering inventions, the rest represented the use of previously known idea or concept but in a novel way [2]. Thus, the conclusion was that an idea of a design solution to new problem might be already known. But where this idea could be found? TRIZ, based on a systematic view of technological world, provides techniques and tools, which help designers to create a new design idea and avoid numerous trails and errors during a problem solving process.
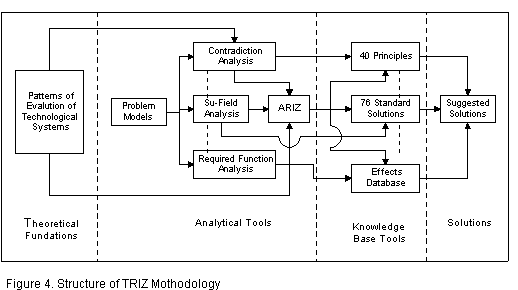
Any problem solving process involves two components: the problem itself and the system in which the problem exists. Successful innovative experience shows that both problem analysis and system transformations are important to problem solving. Accordingly, TRIZ methodology includes the analytical tools for problem analysis, the knowledge base tools for system changing and their theoretical foundations. Figure 4 illustrates the basic structure of TRIZ.
The Patterns of Evolution of Technological System are the theoretical foundation of TRIZ methodology. These patterns indicate that there exist basic laws for engineering system development, and understanding them enhances ones ability to the design problem solving. There are eight patterns and each pattern consists of several sub-patterns or lines [9].
(1) Stages of evolution of a technological system
(2) Evolution toward increase ideality
(3) Non-uniform development of system elements
(4) Evolution toward increase dynamism and controllability
(5) Increased complexity followed by simplification
(6) Matching and mismatching elements
(7) Evolution toward micro-level and increased use of fields
(8) Evolution toward decrease human involvement
Patterns and their lines serve as “soft equation” or “function” describing the system “life curve” in the evolution space. Based on them, the further configurations of a system can be reliably “calculated or forecasted” if the current system configuration is given.
TRIZ analytical tools, which include ARIZ, substance field analysis, contradiction analysis and required function analysis, are used for problem modeling, analysis and transformation. These analytical tools do not use every piece of information about the product where the problem resides. The way they generalize a specific situation is to represent a problem as either a contradiction, or a substance-field model, or just as a required function realization. ARIZ is such a sophisticated analytical tool that it integrates above three tools and other techniques.
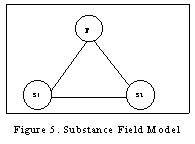
Substance field analysis is a TRIZ analytical tool for building functional model for problems related to existing or new technological systems. Each system is created to perform a certain function. Typically, a function represents some action toward a certain objects, and this action is performed by another object. This situation can be modeled by a triangle whose corners represent objects and an action or interaction (called a field). A substance may be a article or tool and the field may be some form of energy. In general, any properly functioning system can be modeled with a complete triangle as shown in figure 5. Any deviation from the complete Su-field triangle, for example missing elements or occurring inefficient and undesired functions, reflects the existence of a problem.
Contradiction Analysis is a powerful tool of looking problem with the new perspective. In TRIZ standpoint, a challenging problem can be expressed as either a technical contradiction or a physical contradiction. A technical contradiction might be solved by using contradiction table that identifies 39 characteristics most frequently involved in design process. A physical contradiction might be solved by separation principles. Contradiction analysis is the fundamental step to apply 40 inventive principles, one of the knowledge base tools.
Required function analysis refers to select the objective of the system and match it with the function list in the TRIZ Effect Knowledge Base. Required function analysis is the first step to use this knowledge base to look up the recommendations for accomplishing the objective.
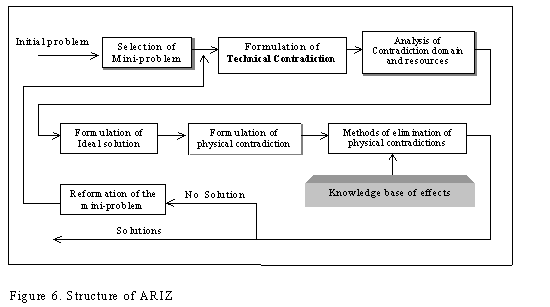
ARIZ refers to Algorithm for Inventive Problem Solving, a set of successive logical procedures directed at reinterpretation of a given problem. In TRIZ standpoint, a technological problem becomes an invention one when a contradiction is overcome. However, “real world” problems do not always appear as contradictions. Furthermore, Su-field analysis and required function analysis may not be applied directly in some situations. Thus it is not obvious how or where to apply TRIZ knowledge base tools to aid the problem solving. ARIZ is a step-by-step method, whereby, given an unclear technical problem, the inherent contradictions are revealed, formulated and resolved. Figure 6 is the structure of ARIZ [5].
TRIZ knowledge base tools include 40 Inventive Principles, 76 Standard Solutions and Effect Database. These tools are developed based on the accumulated human innovation experience and the vast patent collection. The knowledge base tools are different from analytical tools in that they suggest the ways for transforming the system in the process of problem solving while analytical tools help change the problem statement [7].
Forty Inventive Principles are used to guide the TRIZ practitioner in developing useful “concepts of solution” for inventive situation. Each of solution is a recommendation to make a specific change to a system for the purpose of eliminating technical contradictions. Contradiction table recommends which principles should be considered in solving approximately 1250 contradictions.
Seventy-six Standard Solutions were developed for solving standard problems based on the Patterns of Evolution of Technological Systems. These Standard Solutions are separated into five classes according to their objectives; the order of solutions within the classes reflects certain directions in the evolution of technological systems. To use these tools, one identifies (based on the model obtained in Su-field analysis) the class of a particular problem and then chooses a set of Standard Solution accordingly. The standard solution is a recommendation as to what kind of system transformation should be made to eliminate the problem.
Effect Knowledge Base is probably the most easy to use tool in TRIZ. Very early in his research, Altshuller recognized that given a difficult problem, the ideality and ease of implementation of a particular solution could be substantially increased by utilizing various physical, chemical and geometric effects, thus a large vast of database has been developed. In applying Effect Knowledge Base tool, one has to select a appropriate function the system wants to perform (based on the required function analysis), then the knowledge base provides many alternatives for delivering the function.
The following table summarizes the possible relations between Axiomatic Design rules and TRIZ problem solving tools.
Axiomatic Design |
TRIZ |
|
Corollary 1 (Decoupling of Coupled Design)
Decouple or separate parts or aspects of a solution if FRs are coupled or become interdependent in the proposed design.
This corollary states that functional independence must be ensured by decoupling if a proposed design couples the functional requirements. Decoupling does not necessarily imply that the system has to be broken into two or more separate physical parts, or that a new element has to be added to the existing manufacturing system design. Functional decoupling may be achieved without physical separation. However, in many cases, such physical decomposition may be the best way of solving the coupling problem.
|
Contradiction in an engineering system in TRIZ is similar to the functional coupling in AD theory. Overcoming contradiction means the removal of functional coupling in AD.
There are two types of contradictions: technological contradiction and physical contradiction. A technological contradiction is derived from a physical contradiction. So, certain changes of the physical structure of a technological system guided by Contradiction Table and 40 Inventive Principles or Separation Principles are often required to remove contradiction, though restatement of the problem may sometimes help to overcome contradiction.
|
|
Corollary 2 (Minimization of FRs)
Minimize the number of functional requirements and constraints.
Corollary 2 states that as the number of functional requirements and constraints increases, the system become more complex and thus the information content is increased. So, this Corollary recommends the designer strive for maximum simplicity in overall design or the utmost simplicity in physical and functional characteristics.
|
Ideal Final Result (IFR) philosophy corresponds to the Corollary 2 in AD.
IFR states that a system is a “fee” for realization of the required function and IFR will be realized if the system does not exist, but the required function is performed. IFR helps an engineer to focus on concepts that minimize requirements in substance, energy and complexity of engineering product and process. |
|
Corollary 3 (Integration of Physical Parts)
Integration design features into a single physical process, device or system when FRs can be independently satisfied in the proposed solution.
Corollary 3 states that the number of physical components should be reduced through integration of parts without coupling functional requirements. However, mere physical integration is not desirable if it results in an increase of information content or in a coupling of functional requirements.
|
Evolution Pattern 5, Increased Complexity followed by Simplification, corresponds to Corollary 3.
This pattern states that technological systems tend to develop first toward increased complexity (i.e., increased quantity and quality of system functions) and then toward simplification (where the same or better performance is provided by a less complex system).
Line Mo-Bi-Poly reflects that Mono-function products evolve into bi-function or poly-function products through integration of physical embodiments. It is obvious that this integration should not result in a technical contradiction, that is a coupling.
|
|
Corollary 4 (Use of Standardization)
Use standardization or interchangeable parts if the use of these parts is consistent with FRs and constraints.
The corollary states a well-known design rule: use standard parts, methods, operations and routine, manufacture, and assembly. Special parts should be minimized to decrease cost. Interchangeable parts allow for the reduction of inventory, as well as the simplification of manufacturing and service operations, i.e., they reduce the information content.
|
No Patterns, principles or tools correspond to this corollary. TRIZ focus its studies on inventive problem solving, so it pays less attention to the standardization and interchangeability of physical components.
|
|
Corollary 5 (Use of Symmetry)
Use symmetrical shapes and/or arrangements if they are consistent with the FRs and constraints.
It is self-evident that symmetrical parts are easier to manufacture and easier to orient in assembly. Not only should the shape be symmetrical wherever possible, but hole location and other features should be placed symmetrically to minimize the information required during manufacture and use. Symmetrical parts promote symmetry in the manufacturing process.
|
Principle 4, Asymmetry (one of 40 Inventive Principles) in TRIZ is in opposition to Corollary 5 in Axiomatic Design.
The reason why TRIZ and AD offer opposite principles is that AD theory states the general rules of engineering design, but TRIZ methodology concentrates its studies on the inventive problem solving techniques. These techniques were derived from the patent database, which relates to novel methods and unique ideas.
|
|
Corollary 6 (Largest Tolerance)
Specify the largest allowable tolerance in stating functional requirements.
This corollary is a consequence of both Axiom 1 and Axiom 2. Since it becomes increasingly difficult to manufacture a product as the tolerance is reduced, more information is required to produce parts with tight tolerances. On the other hand, if the tolerance is too large, then the error in assembly may accumulate such that FR cannot be satisfied. Therefore, the tolerance should be made as large as possible, but should remain consistent with the likelihood of producing functionally acceptable part.
|
No corresponding tools are found in TRIZ.
Corollary 6 is a general rule of design and it is nothing to do with invention.
|
|
Corollary 7 (Uncoupled Design with less Information)
Seek an uncoupled design that requires less information than coupled designs in satisfying a set of FRs.
This corollary is a consequence of Axiom 1 and 2. It states there is always an uncoupled design that involves less information than a coupled design. The implication of this corollary is that if a designer proposes an uncoupled design which has more information content than a coupled design, then the designer should return to the “drawing board” to develop another uncoupled or decoupled design having less information content than the coupled design.
|
40 Inventive Principles and Line of Mo-Bi-Poly.
40 Inventive Principles provide the techniques to overcome contradictions.
Evolution Line “Mo-Bi-Poly” offers guidelines to reduce the complication of a system.
|
|
Theorem 1 (Coupling Due to Insufficient Number of DPs)
When the number of DPs is less than the number of FRs, either a coupled design result or the FRs cannot be satisfied.
Theorem 2 (Decoupling of Coupled Design)
When a design is a coupled due to the greater number of FRs than DPs (m>n), it may be decoupled by the addition of the design new DPs so as to make the number of FRs and DPs equal to each other, if a set of the design matrix containing n´n elements constitutes a triangular matrix. |
Substance Field Analysis states any properly functioning system can be modeled with a complete Su-field triangle and any deviation from a “complete” triangle, for example missing one element, reflects the existence of a problem.
Building a Su-field Model, one of 76 Standard Solutions, shares the same idea with Theorem 2 in AD. This Standard Solution states: if a given object is unreceptive (or barely receptive) to required changes and the problem description does not include any restriction for introducing substances or fields, the problem can be solved by completing the Su-field model to introduce the missing element.
|
|
Theorem 5 (Need for New Design)
When a given set of FRs is changed by the addition of a new FR, or substitution of one of the FRs by a new one, or by selection of a completely different set of FRs, the design solution given by original DPs cannot satisfy the new set of FRs. Consequently, a new design solution must be sought.
|
Enhancing Su-field Model, Class 2 of 76 Standard Solutions, corresponds to Theorem 5.
The addition of a new FR, or substitution of one of the FRs by a new one means the previous system is an inefficient Su-field model, i.e., the system is not effective enough. In this case, enhancing Su-field model is required to improve the system functions.
|
1. The basic premise of the axiomatic approach to design is that there are basic principles that govern decision making in design, just as the laws of nature govern the physics and chemistry of nature. Two basic principles, Independence Axiom and Information Axiom, are derived from the generation of good design practices. The corollaries and theorems, which are direct consequences or are derived from the axioms, tend to have the flavor of design rules.
2. The main axiom of TRIZ is that the evolution of technological systems is governed by objective patterns. These patterns can be employed for conscious development of technological system and inventive problem solving, replacing the inefficiencies of blindly searching. These patterns and other TRIZ tools are revealed by analysis of hundreds and thousands of inventions available in the world patent database.
3. Axiomatic design pays much attention to the functional, physical and process hierarchies in the design of a system. At each layer of the hierarchy, two axioms are used to assess design solutions. However, TRIZ abstracts the design problem as either the contradiction, or the Su-field model, or the required function realization. Then corresponding knowledge base tools are applied once the problem is analyzed and modeled. Though approaches to the solutions are of some differences, many design rules in AD and problem-solving tools in TRIZ are related and share the same ideas in essence.
1. Suh, N.P., “The Principles of Design”, Oxford University Press, 1990
2. G.S. Altshuller, “Creativity as an Exact Science”. Gordon and Breach Science Publishers, 1984
3. Leonard D.A and Suh N.P “Axiomatic Design and Concurrent Engineering”. Computer-Aided Design, Vol 26, N 7 July 1994
4. G.S Altshuller, “And Suddenly the Inventor Appeared”. Technical Innovation Center, Inc. 1996
5. Victor R.Fey and Eugene I. Rivin, “The Science of Innovation—A Managerial Overview of the TRIZ Methodology”, TRIZ Group,1997.
6. Mann, D.L., “Axiomatic Design and TRIZ: Compatibility and Contradictions” ,TRIZ Journal, June and July 1999
7. Boris Zlotin and Alla Zusman, “Mapping Innovation Knowledge” April 1999, Triz-Journal.
8. Alla Zusman and John Terninko, “TRIZ/Ideation Methodology for Customer Driven Innovation”, Ideation International Inc. 1996.
9. “Tools of Classical TRIZ”. Ideation International Inc. 1999.
10. Invention Machine Lab 2.12.
11. Shinya.S and Kawassaki.U, “A Study of Creative Design Based on the Axiomatic Design Theory”, DE-Vol.68, Design Theory and Methodology-DTM’94 ASTM 1994
12. Nam P. Suh and Shinya Sekimoto “Design of Design Thinking Machine” Ann.CIRP Vol 39 No 1 (1990) pp 145-148